Trigonometrical ratios for related angles

Trigonometrical ratios for related angles

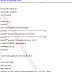
360^o Corresponds to one full revolution.sine of angles of  360^o+45^o;720^o+45^o;1080^o+45^o are equal to sine of 45^o.This is so far the other trigonometrical ratios.That is,When an angle exceeds 360^o,it can be reduced to an angle between 0^o and 360^o by wiping out integral multiples of 360^o

sin(- θ) = -sinθ

sin(90- θ)=cos θ

sin(90+ θ)=cos θ

sin(180- θ)=sin θ

sin(180+ θ)= -sin θ

sin(270- θ)= -cos θ

sin(270+ θ)=-cos θ

sin(360- θ)= -sin θ

cos(- θ) = cos θ

cos(90- θ)=sinθ

cos(90+ θ)= -sinθ

cos(180- θ)= -cos θ

cos(180+ θ)= -cos θ

cos(270- θ)= -sinθ

cos(270+ θ)=sinθ

cos(360- θ)= cos θ

tan(- θ) = -tan θ

tan(90- θ)=cotθ

tan(90+ θ)= -cotθ

tan(180- θ)= -tan θ

tan(180+ θ)= tan θ

tan(270- θ)= cotθ

tan(270+ θ)= -cotθ

tan(360- θ)= -tan θ

cosec(90+ θ)=sec θ

cosec(180- θ)=cosec θ

cosec(180+ θ)= -cosec θ

cosec(270- θ)= -sec θ

cosec(270+ θ)=-sec θ

cosec(360- θ)= -cosec θ

sec(- θ) = sec θ

sec(90- θ)=cosecθ

sec(90+ θ)= -cosecθ

sec(180- θ)= -sec θ

sec(180+ θ)= -sec θ

sec(270- θ)= -cosecθ

sec(270+ θ)=cosecθ

sec(360- θ)= sec θ
 
cot(- θ) = -cotθ

cot(90- θ)=tanθ

cot(90+ θ)= -tanθ

cot(180- θ)= -cot θ

cot(180+ θ)= cot θ

cot(270- θ)= tanθ

cot(270+ θ)= -tanθ

cot(360- θ)= -cot θ